POLAR EYES THE CUSP

ABSTRACT

Measurements by the POLAR spacecraft show that the polar cusp is affected by the angle of the magnetic dipole axis to the direction of the solar wind flow, the dynamic pressure of the solar wind and the direction of the interplanetary magnetic field. The polar cusp moves equatorward with increasing southward interplanetary magnetic field and much less, but also in the equatorward direction, for increasingly northward magnetic field. The cusp moves to the afternoon side for positive Y components of the interplanetary magnetic field in the northern hemisphere and toward dawn in the southern hemisphere. Negative Y components, in the direction of the Earth's orbital motion, have the reverse effect on the cusp position. Higher dynamic pressure extends the cusp to greater local times away from noon and thickens it in the poleward direction. When the IMF is nearly due northward, reconnection tailward of the cusp can occur producing magnetosheath plasma on magnetic field lines that have two feet in the ionosphere. This process transfers momentum to the magnetospheric plasma, and creates the low-latitude boundary layer. Near the cusp the magnetic field lines connected to the Earth reverse rapidly across a thin current sheet that could be described as the magnetopause. However, there is a second current sheet further out, beyond which field lines are no longer connected to the ionosphere. D-shaped particle distributions in this region are consistent with reconnection tailward of the cusp. The distributions evolve to be more isotropic with time since reconnection. Perhaps surprisingly, the magnetosheath plasma moving toward the cusp reconnection site is slowed greatly on the field lines connected to the ionosphere.

INTRODUCTION

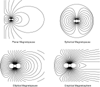
The earliest model of the polar cusp appears in Chapman and Ferraro's image dipole model of the magnetopause [Chapman and Ferraro, 1930]. In this model an unmagnetized solar wind intercepts the Earth's dipolar magnetic field. The magnetic field is devoid of plasma. The solar wind is assumed to arrive as a planar slab whose affect on the magnetic field can be modeled as a mirrored dipole of equal magnitude and a parallel direction upstream from the planar magnetopause at the same distance from the magnetopause as the Earth's dipole. A "magnetosphere" of this type is shown in Figure 1 on the upper left. In this model the unmagnetized solar wind penetrates down to the ionosphere in a narrow funnel which today we would call the polar cusp.

Figure 1. Four models of the magnetosphere. (Upper left) Chapman and Ferraro's [1930] image dipole model. (Upper right) A dipole field in a spherical boundary. (Lower left) A dipole field in an elliptical cylinder. (Lower right) The empirical model of Tsyganenko [1989] based on the observed magnetosphere that contains plasma.

The location of the polar cusp depends on the shape of the magnetopause as is illustrated by the spherical magnetopause shown in the upper right, with its cusp directly over the pole and the elliptical magnetopause on the lower left. It also is affected by the presence of plasma in the magnetosphere as illustrated by the empirical model of the magnetosphere of Tsyganenko [1989] shown in the lower right.

A further complication is that the solar wind plasma is magnetized. In a collisionless plasma, charged particles that are on a magnetic flux tube stay with that flux tube under ordinary plasma conditions. Thus it is difficult to understand how the solar wind plasma can leave its magnetic flux tubes and enter those of the polar cusp. Many theories have been proposed for this entry. Waves and turbulence can cause particles to cross magnetic field lines if they resonate with the motion of the charged particle. They can also cross the magnetic field or, equivalently, field lines can change partners at some point, if there is a parallel electric field. Then the problem becomes how to create that electric field along the magnetic field. Again waves may play a role in creating this parallel electric field, but other processes are possible as well.

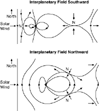
The process by which tubes of magnetized plasma switch partners is called reconnection. Dungey [1961; 1963] used this process to create a model of the magnetosphere that not only produced a polar cusp containing solar wind plasma but also was able to drive a circulation of plasma in the magnetosphere. As illustrated in the upper panel of Figure 2, when the interplanetary magnetic field (IMF) was southward, the plasma flow moved over the poles to the tail. There the magnetic field reconnected again and returned to the dayside of the magnetosphere to possibly repeat the cycle once again. This process was able to transfer mass, momentum and energy to the magnetosphere and has been used to explain many of the dynamic processes within the magnetosphere including both storms [Burton et al., 1975] and substorms [Russell and McPherron, 1973]. When the IMF was due northward, Dungey proposed the model illustrated in the lower panel of Figure 2. Here reconnection occurs tailward of the cusp and closed flux tubes containing solar wind plasma are added to the dayside. This also sets up a magnetospheric circulation pattern with closed flux tubes moving tailward around the magnetosphere at low latitudes but this process seems not to couple as much momentum from the solar wind as the southward mechanism. This may be because the reconnection region is smaller and that it couples slowly moving plasma to the magnetosphere, rather than the swiftly moving plasma of southward reconnection. Moreover, when the IMF is southward, magnetic energy is expended in the acceleration of the plasma at the nose.

There is one other difference between the two Dungey models. Even though both models create a polar cusp, northward reconnection creates it equatorward of the bifurcation field line while the southward reconnection model creates the polar cusp poleward of the reconnection line. The bifurcation field line is the field line that divides the magnetic flux that bends equatorward from the flux that bends tailward.

Figure 2. Dungey's [1961; 1963] two models of the reconnecting magnetosphere for southward (top) and northward (bottom) IMF.

Several missions have explored the high altitude polar magnetosphere before POLAR. The first to do so was the European HEOS 2 [Haerendel et al., 1978]. For almost two decades much of our understanding of the polar cusp, and the mantle was derived from the observations of this spacecraft. However, this understanding was limited by the relatively long orbital period of the spacecraft that took it through the polar magnetosphere relatively seldom and also by a low data rate that did not allow for rapid sampling of the plasma. This was followed many years later by the US mission Hawkeye that also had a long period and a low sampling rate [Farrell and Van Allen, 1990; Van Allen, 1992]. Several problems led to the main analysis of the data not taking place until the 1990's [Zhou and Russell, 1997; Fung et al., 1997]. Other satellites such as OGO-5 and IMP-5 with moderate inclinations provided occasional entries into the polar cusp at high altitudes [Russell et al., 1971; Frank, 1971]. Most of our understanding of the cusp in fact is based on the thousands of cusp encounters observed by lower altitude missions such as the DMSP satellites, Dynamics Explorers etc. The observations of these spacecraft will be used in this review to compare and contrast with the results at high altitudes.

CUSP IDENTIFICATION

On the POLAR spacecraft the polar cusp is identified by its signatures in the thermal electron and ion density and temperature, the ion composition and the magnetic field. Figure 3 shows these signatures for IMF directions that are northward, southward, eastward and westward [Zhou et al., 1999a]. The ion densities shown here are partial densities over restricted energy ranges so they do not always equal the electron densities. The densities generally increase in the cusp well above the neighboring values and the temperature of the plasma is generally cool relative to that of the magnetospheric plasma. The ion composition reflects that of the magnetosheath with about 5% He⁺⁺. The signature that we will use to delimit the boundaries of the cusp is the magnetic field strength as compared with a model of the magnetic field expected in this region [Tsyganenko, 1996]. The magnetic field is depressed in this region and becomes very noisy as shown in each of the four panels of Figure 3. The decrease in the magnetic field strength reflects a decrease in the magnetic field pressure that compensates for the increased thermal pressure of the cusp plasma. Because magnetic pressure is proportional to the square of the magnetic field, the decrease in energy of the magnetic field in the cusp is measured by the product of the background field strength times the decrease in the field. The plasma density and the plasma pressure are relatively constant with altitude along the cusp. Hence the magnetic depression associated with the cusp weakens as the background field increases. At low altitudes the magnetic signature is virtually absent but at high altitudes above about 5 R_E, the magnetic field provides a good signature of the presence of polar cusp plasma.

Figure 3. Parameters used to identify high altitude polar cusp crossings in the study of POLAR data by Zhou et al. [1999a]. The top panel shows the observed magnetic field less the model field of Tsyganenko. Below are the electron density and temperatures measured by the Hydra sensors and the partial density of protons and their energy measured by the TIMAS sensors.

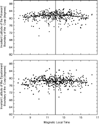
Using these signatures we have identified the location of the polar cusp over the first two years of the POLAR mission. These positions are plotted in Figure 4 for the poleward and equatorward edges of the cusp on the top and bottom of the plot respectively. The average location of the cusp is between 78^o and 81^o near noon and droops to slightly lower invariant latitudes toward dawn and dusk. There is much scatter in the individual measurements because the cusp location is sensitive to many external factors such as the direction of the IMF and the tilt of the dipole. The IMF effect is not necessarily instantaneous if the plasma is being eroded and carried tailward but rather the cusp moves somewhat slowly. Moreover when the satellite encounters the cusp it may be in transit from one equilibrium location to another and that is observed at a place different than the location expected for the solar wind condition in effect even for those effects that take place rather rapidly. Thus we make our conclusions based on measures such as the medians shown in Figure 4 and least-square fits to the data.

Figure 4. The invariant latitude of the polar cusp as a function of magnetic local time. Top, polar edge; bottom, equatorward edge [Zhou et al., 1999a].

EFFECT OF THE TILT OF THE DIPOLE

The tilt of the dipole is the complement of the angle that the dipole axis makes with the nominal solar wind direction, which is usually taken to be the direction away from the sun. The optimum definition would include the 29.5 km/s orbital velocity of the Earth and the true solar wind direction that can be non-radial especially after the passage of an interplanetary shock. However, here we use just the GSE X direction.

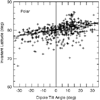
Figure 5 shows the locations of the center of the polar cusp versus the tilt angle of the dipole. All POLAR observations in this study were obtained in the northern hemisphere. When the tilt angle is negative, the northern dipole axis is pointed away from the sun and when it is positive, the dipole points toward the sun. We see that the best fit line indicates that the cusp location changes about 5^o for a tilt change of 70^o or about 1^o invariant latitude for every 14^o of tilt [Zhou et al., 1999b]. A similar dependence is seen in the DMSP cusp position at low altitudes [Newell and Meng, 1989]. This dependence means that the polar cusp is usually at different invariant latitudes in the northern and southern hemispheres and especially at the solstices when the tilt angle is the greatest.

Figure 5. The invariant latitude of the center of the cusp versus dipole tilt angle [Zhou et al., 1999b]. Solid bars show median values.

The IMF is thought to control where reconnection occurs on the magnetopause. In the Dungey model southward IMF leads to reconnection at the subsolar point and northward IMF leads to reconnection behind the polar cusp. However, the IMF By component can play a role too, sliding the reconnection site around the magnetopause to a location most favorable for reconnection [Crooker, 1979; Luhmann et al., 1984] such as when the magnetic field in the magnetosheath and the magnetosphere are nearly antiparallel. Thus we will examine the effects of both components on the cusp location.

Figure 6 shows the dependence of the invariant latitude of the center of the cusp on the north-south component of the IMF [Zhou et al., 1999a]. An increasingly southward magnetic field makes the cusp move equatorward. An increasingly northward IMF has a much weaker effect. In fact the cusp moves slightly equatorward for increasing northward IMF. Perhaps surprisingly this is as expected for the Dungey northward model because the magnetosheath plasma enters the magnetosphere equatorward of the bifurcation line and the stronger is the rate of northward reconnection the wider in latitude and more equatorward we might expect the cusp to be. In any case this effect is small and implies that reconnection while present does not much affect the transport of plasma when the IMF is northward.

Figure 6. The invariant latitude of the polar cusp versus the IMF Bz component [Zhou et al., 1999a].

These results are qualitatively in accord with those at low altitudes [Newell et al., 1989] but only qualitatively. In this DMSP study the cusp is displaced further equatorward for southward IMF and continues to move poleward for increasingly northward IMF. We attribute these differences to the use of hourly averages in the DMSP studies as opposed to instantaneous values for POLAR studies.

The Dungey model is for strictly northward and southward IMF and, although we expect some differences for By finite in the circulation of plasma [Russell, 1972], we expect qualitatively similar behavior for all southward IMF cases and all northward cases as two groups. Nevertheless it is prudent not to attempt to separate the data into two classes according to an infinitesimal component because of fluctuations in the solar wind flow direction, effects of the magnetosheath on the field and flow and natural fluctuations in the magnetic field and flow. Thus we will divide the data into three groups: northward IMF with angles of the field more than 15^o above the magnetospheric equator as projected in the Y-Z plane; southward IMF fields 15^o below the equatorial plane; and horizontal fields. This divides the data into three roughly equal sets and keeps northward and southward behavior strictly separate.

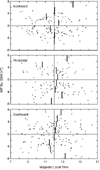
Figure 7 shows the magnetic local time where the cusp was encountered as a function of the IMF By component for each of our three categories: north, horizontal and south fields. Since the cusp is broad in local time as shown in Figure 4, we expect the wide scatter in the encounter locations that we see. However, if the IMF By pulls the cusp to the side, we might expect a shift in the median position with changing By. We do not see such a shift for northward or horizontal fields but there is a significant shift with increasing By in the bottom panel for southward IMF. The cusp moves toward the afternoon side for increasingly positive By and toward the morning side for increasingly negative By. This is most easily interpreted in terms of a shifting reconnection site as conjectured by Crooker [1979] and is seen in simulations [Russell, 1999]. Such behavior is also seen at low altitudes in the DMSP results that also confirm that the reverse behavior is seen in the southern hemisphere [Newell et al., 1989]. This result is somewhat surprising because the reconnection site on the magnetopause must split into two regions, one on the morning side and one on the evening side, if the northern and southern hemispheres see opposite shifts, but when reconnection occurs it should form a pair of lines (at each reconnection site), one connected to the north and one to the south at the local time of the reconnection site. Hence we might expect the cusp to split into two and not to shift in opposite ways in local time in each hemisphere. However, it does shift oppositely in the two hemispheres. Simulations show that the short leg of the polar cusp is the one that becomes most developed and cusp-like when the reconnection site on the magnetopause splits and moves poleward and away from noon.

Figure 7. The magnetic local time of the polar cusp versus the IMF By component for northward (top), horizontal (middle) and southward (bottom) IMF [Zhou et al., 1999a].

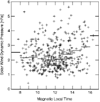
The solar wind dynamic pressure controls the size of the magnetosphere and it has also been shown to have a weak effect on geomagnetic activity. Thus it might also have some effect on the location and properties of the cusp. We have found two such effects. The first of these is shown in Figure 8 that shows that the times when the cusp is found at local times far from noon are times of greater solar wind dynamic pressure. This indicates that the cusp extends to earlier and later local times when the solar wind presses harder on the magnetosphere [Zhou et al., 1999a].

Figure 8. The median dynamic pressure at each local time when the polar cusp was observed [Zhou et al., 1999a].

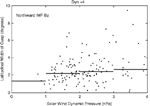
The second effect is shown in Figure 9. The cusp has a greater width in invariant latitude for higher dynamic pressure [Zhou et al., 1999a]. We have purposefully restricted the range of the dynamic pressure to less than 4 nPa to avoid situations in which the spacecraft approaches the magnetopause where the invariant latitude of the cusp is difficult to calculate.

Figure 9. The width of the cusp in invariant latitude versus solar wind dynamic pressure [Zhou et al., 1999a].

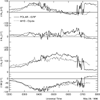
Figure 10. The observed magnetic field minus the IGRF magnetic field for the May 29, 1996 polar cusp encounter together with the MHD simulation minus the dipole field [Russell et al., 1998].

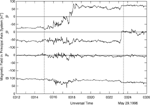
When the IMF is strongly northward, reconnection occurs tailward of the polar cusp and adds magnetosheath plasma to the dayside magnetosphere. This process is observed by POLAR and is consistent with simulations that can very well simulate the magnetic field and plasma conditions observed [Russell et al., 1998]. This is shown in Figure 10 that compares POLAR magnetic field observations with the computer simulations of Fedder et al. [1997] for the solar wind conditions of May 29, 1996. There is excellent agreement except near the end of the interval where POLAR sees a sharp exit from the distorted field while the simulation shows a more gradual change. Moreover, POLAR moved back and forth through a rather sharp current layer several times before making its final exit into the tail-like field region on the right. This suggests to us that the current layer that separates the field lines pointing northward toward the reconnection point on the exterior of the magnetosphere are separated from the returning field lines closer to the Earth by a rather thin current layer. This layer is perhaps best called the magnetopause current but perhaps it is better to think of the entire region of field change as the magnetopause. Figure 11 shows the magnetic field even closer to the reconnection point seen by Interball earlier on this day as it crossed this current layer at 0317 UT and then later at 0322:30 crossed a second current layer and entered the flowing magnetosheath plasma with an entirely new magnetic field direction [Savin et al., 1998]. Thus near the cusp the magnetic field lines connected to the ionosphere on the dayside are pulled up in a Vee-shaped fashion covering the usual polar cusp entry funnel. Then fast magnetosheath flow is outside this region and there are two district current layers one on the boundary between the sheath flow and the more stagnant plasma in the process of being incorporated into the magnetosphere.

Figure 11. The magnetic field observed by Interball as it crossed through the region of the high latitude magnetopause on May 29, 1999 when the IMF was strongly northward [Savin et al., 1998].

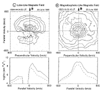
This field configuration shown in the panel at the top of Figure 12 illustrates the magnetic field configuration inferred for June 20, 1996 [Russell et al., 1999]. The magnetic field seen by POLAR and the solar wind dynamic pressure are seen in the lower part of the figure. During this pass the solar wind dynamic pressure was increasing and we believe the spacecraft moved from point L to M in this figure at the times marked L and M. Figure 13 shows the ion measurement of TIMAS at times L and M in a display of the distribution function versus parallel and perpendicular velocity. The L distribution has a magnetosheath-like particle beam with almost zero velocity across the field in the upper half of the plot. This is D-shaped and indicates slowed magnetosheath flow entering the magnetosphere. The beam with negative parallel velocities consists of energetic ions that have entered the magnetosphere and been reflected by converging magnetic fields close to the ionosphere. We interpret this distribution as one occurring on a field line that has

Figure 12. Observations of the polar cusp on June 20, 1996 and their interpretation. The top panel sketches the location of POLAR relative to the cusp geometry prior to the increase in dynamic pressure. The dynamic pressure increase shown in the bottom panel is supposed to move the cusp to point L and their point M. The middle panels show the magnetic field observed and as modeled by Tsyganenko [1996]. See Russell et al. [1999].

Figure 13. Observations of the angular distribution function observed by TIMAS on June 20, 1996 [Fuselier et al., 1999]. /TD>

just recently reconnected. Its direction indicates that it is on the Earthward side of the current sheet that leads to the reconnection site. The isotropic distribution is an older flux tube that reconnected much earlier than the field line on which the first distribution was found. This distribution appears to have isotropized. This isotropization is possible since, for this orientation of the IMF, the field lines though both L and M may be connected to the Earth on both ends. Similar diagrams and distributions can be drawn for other events too [Russell et al., 1999]. Recently these same distributions have been interpreted as reconnection equatorward of the cusp for northward IMF by Fuselier et al. [1999]. We believe that our explanation is much simpler than theirs and that it agrees with all previous understanding of the effect of the IMF and the magnetosphere.

POLAR high altitude measurements have allowed us to test many of the basic conjectures of the behavior of the high-latitude magnetosphere and also to test MHD simulation models in a critical region. The observations strongly support the ideas of Dungey [1961; 1963] on how the IMF controls reconnection and the entry of mass momentum and energy into the magnetosphere. The MHD simulations appear to be very useful on the grand or global scale but do not predict the observed thin current sheet near the cusp. However, there clearly is a thin current sheet causing a field reversal and a second exterior current sheet bounding the flowing magnetosheath plasma from the more stagnant plasma. All the evidence is that reconnection occurs as Dungey envisioned it in this region although he did not attempt to sketch the region to scale.

The polar cusp is a very turbulent region as suggested by Figure 13. What is not evident in this figure is that much of the turbulence in the cusp is due to ion cyclotron waves. This study is still in progress and will be reported later.

The role of the IMF is also seen in the behavior of the invariant latitude of the cusp in response to the north-south component and the motion of the cusp in local time in response to the By component. These are all expected to occur due to reconnection. Since reconnection appears to explain the transfer of plasma from the magnetosheath field to the magnetospheric field, one then questions whether the other mechanisms that have been postulated for this transfer are at all effective or necessary. While it is beyond the scope of this review to examine all such previous claims, we know of no observations from POLAR that require any process other than reconnection to explain the solar wind entry.

This leaves open the question though of what causes reconnection. Progress is being made in understanding this process both theoretically and observationally [Shay et al., 1999; Scudder et al., 1999]. The answer appears to be found on the smallest scales below the ion gyro radius where the ions become unmagnetized but the electrons remain magnetized. This allows the electric field build up along the magnetic field to take place without the need to involve waves or turbulence.

Of course the magnetosphere also responds to the normal stresses of the solar wind. We see this response in the variation of the location of the cusp with dipole tilt angle and the breadth and thickness of the high altitude cusp with increasing dynamic pressure. Nevertheless most of the tangential stress on the magnetosphere appears to be provided by the reconnection process and it is important that we an understand it. Through the efforts of theorists, numerical modelers and observationalists, we now are very close to such an understanding.

I would like to express my gratitude to the numerous colleagues who have worked with me over the years in studying the magnetosphere. I have learned much from them. In particular on the analysis of the POLAR data I have benefited greatly from working with Jack Scudder, Joel Fedder, Steve Fuselier, X-W. Zhou, Frances Fenrich, Sergey Savin, Guan Le, Janet Luhmann. Nickolai Tsyganenko, Cindy Cattell and Pat Reiff. This work was supported by the National Aeronautics and Space Administration under research grant NAG5-7721.

Burton, R.K. et al. 1975, J. Geophys. Res., 80, 4204-4214

Chapman, S., and Ferraro, V.C.A. 1930, Nature, 126, 129

Crooker, N.U. 1979, J. Geophys. Res., 84, 951-959

Dungey, J.W. 1961, Phys. Rev. Lett., 6, 47-48

Dungey, J.W. 1963, in Geophysics: The Earth's Environment, edited by C. Dewitt, J. Hieblolt, and A. Lebeau, Gordon and Breach, New York, pp. 505-550

Farrell, W.M. and Van Allen, J.A. 1990, J. Geophys. Res., 95, 20,945-20,958

Fedder, J.A. et al. 1997, Geophys. Res. Lett., 24, 2491-2494

Frank, L.A. 1971, J. Geophys. Res., 76, 5202-5219

Fung, S.F. et al. 1997, Physics and Chemistry of the Earth, 22, 653-662

Fuselier, S.M. et al., 1999, J. Geophys. Res., accepted

Haerendel, G. et al. 1978, J. Geophys. Res., 83, 3195-3216

Luhmann, J.G. et al. 1984, J. Geophys. Res., 89, 1739-1742

Newell, P.T. and Meng, C.-I. 1989, J. Geophys. Res., 94, 6949-6953

Newell, P.T. et al. 1989, J. Geophys. Res., 94, 8921-8927

Russell, C.T. 1972, in Critical Problems of Magnetospheric Physics, Proceedings of the Joint COSPAR/IAGA/URSI Symposium, edited by E. R. Dyer, IUCSTP Secretariat, National Acad. of Sci., Washington, D. C. , pp. 1-16

Russell, C.T. 1999, Adv. Space Res., in press

Russell, C.T. and McPherron, R.L. 1973, Space Sci. Rev., 15, 205-266

Russell, C.T. et al. 1971, J. Geophys. Res., 76, 6743-6764

Russell, C.T. et al. 1998, Geophys. Res. Lett., 25, 3015-3018

Russell, C.T. et al. 1999, J. Geophys. Res., submitted

Savin, S.P. et al. 1998, Geophys. Res. Lett., 25, 2963-2966,.

Scudder, J.D. et al. 1999, J. Geophys. Res., to be submitted

Shay, M.A. et al. 1999, Geophys. Res. Lett., 26, 2163-2166

Tsyganenko, N.A. 1989, Planet. Space Sci., 37, 5-20

Tsyganenko, N.A. 1996, in Proceedings of the ICS-3 Conference on Substorms, ESA SP-389, ESA, Paris, pp. 181-185

Van Allen, J.A.,1992, J. Geophys Res., 97, 6381-6395

Zhou, X.-W. and Russell, C.T. 1997, J. Geophys. Res., 102, 105-110

Zhou, X.-W. et al. 1999a, J. Geophys. Res., in press

Zhou, X.-W. et al. 1999b, Geophys. Res. Lett., 26, 429-432

Back to CT Russell's page More On-line Resources
Back to the SSC Home Page